相对湿度: To Average, or Not to Average?

Lowe (1976)¹描述了以下用于计算饱和蒸气压的模型：

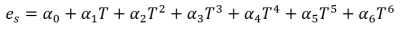
在哪里T.温度在摄氏度和装有系数的值（α） 如下面所述：

While there are other methods to obtain saturation vapor pressure that we could consider, the Lowe model is used in Campbell Scientific data loggers, so I’ll limit my discussion to that method for the purposes of this article.

T.here are two reasons why a simple average of RH over a given data interval is generally not the best practice. First, RH is a calculated value. Mathematicians and statisticians advise us that it is better to use the original data in operations such as averages, then calculate the desired value. In addition, RH is, by definition, limited to values between zero and 100. Therefore, values near those upper and lower bounds cannot vary as much as those toward the middle, which violates statistical assumptions.

T.he second and more important reason not to use simple averaging for RH is that the value can change rapidly with temperature, especially at higher temperatures.图1S.hows the relationship between temperature and saturation vapor pressure based on the Lowe model.

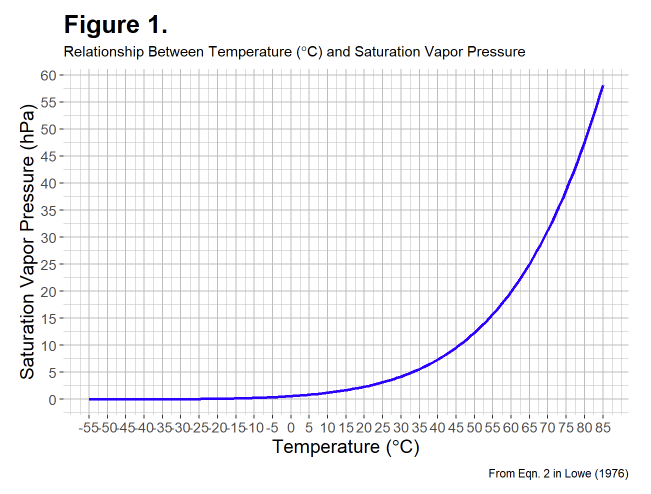
特别注意是饱和蒸汽压力在较高温度下更快地变化。因此，在较高温度下Rh的简单算术平均值可能具有更大的误差。平均超过更长的间隔也可能导致更大的错误。

How to Correctly Average RH

在给定间隔中获得平均RH的优选方法是平均蒸气压和饱和蒸气压力，然后从这些平均值计算RH。这是一个链接download a CRBasic programthat does just that. During each scan, this program calculates vapor pressure and saturation vapor pressure, then stores the vapor pressure averages in a temporary, hidden table. Those values are then used to calculate RH, and all the values are stored in the main hourly table.

为了说明计算RH的平均值的两种方法（简单的算术与平均蒸汽压力）之间的差异，我从犹他州洛根的坎贝尔科学设施附近的气象站收集了大约17个月。使用类似于上面描述的程序，并且数据以四个间隔存储：

• 30 minutes
• 1hour
• 3小时
• 24 hours

图2.S.hows the results:

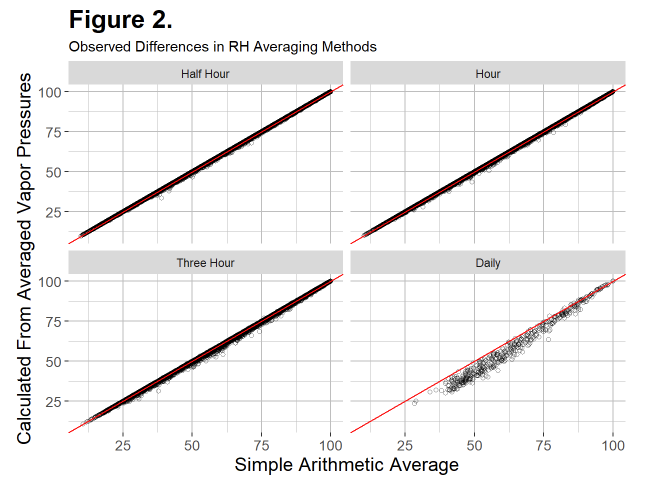
如果这两种方法是一样的,我们所期望的all the datapoints to line up on the red 1:1 lines. For the half-hour and hourly intervals, there is good agreement. However, for longer intervals, error increases substantially. Interestingly, at least in these data, error due to simple averaging nearly always results in a positive bias. In other words, a simple arithmetic average almost always overestimates the mean relative humidity.

世界气象组织（WMO）²和American Association of State Climatologists (AASC)同时建议不确定性(RH错误)measurement be less than 3%. In the worst case, error due to averaging RH would be additive to the sensor error. As an example, if a sensor specification states that it is within 2.3% over most of the range, any additional error would need to be limited to less than 1% to conservatively meet the WMO and AASC recommendations.

表格1显示了一些统计差异的平均方法（简单平均减去蒸气压平均值），包括平均值，最大值和最小值，以及一些百分比。50％的数据位于25和75个百分位之间，95％的数据位于2.5和97.5百分位之间。

表格1.简单平均值与平均蒸汽压力之间的差异比较

对于半小时和每小时间隔，大多数数据均为彼此的1％。因此，根据您使用的传感器以及数据的目的，这些间隔可以接受简单的平均值，以及其他考虑因素。然而，更大的间隔显示出大量偏差，并证明简单的RH平均值不会呈现良好的数据。此外，值得注意的是，对于许多数据来存储其他度量，例如露点或简单地，蒸气压，它可能更具信息量。

我希望这有助于澄清为什么对数学和统计的平均相对湿度以及务实的原因，这通常不是一个好主意。如果您真正需要平均RH，特别是超过较长的时间间隔，更好的做法是使用平均观察和饱和蒸汽压力来计算每次间隔的RH。欢迎你修改可下载的示例程序as needed. Alternatively, simply store the observed vapor pressure and temperature (easily done in a Campbell Scientific data logger). From these, both the relative humidity and dewpoint can be calculated in post-processing if needed.

如果您对平均相对湿度（RH）有任何疑问，请在下面发布。